画像をダウンロード (2√3-5√2) and (√3 2√2) add 271583-Add 2 root 3 - 5 root 2 and root 3 + 2 root 2

 Solve x^4 – 5x^3 4x^2 8x – 8 = 0 Given (1 – √5) is a root of the polynomial equation asked in Theory of Equations by Aryan01 ( 502k points) The product 3√2 4√2 12√32 equals to (a) √2 (b) 2 (c) 12√2 (d) 12√32 individually in the form whose index will be equal to LCMसिद्ध कीजिए कि 3 2√5 एक अपरिमेय संख्या है3 2√5 proved by Sunil sirDoston aaj is video me vide

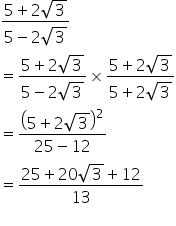
5 2 3 5 2 3 Mathematics Topperlearning Com Rvsvlgmm

Add 2 root 3 - 5 root 2 and root 3 + 2 root 2

Add 2 root 3 - 5 root 2 and root 3 + 2 root 2-21k views asked in Class IX Maths by navnit40 Expert (404k points) Simplify by rationalising the denominator (2 / 5 √3) (2 / 5 √3)Answer (1 of 9) The problem, (√2√3)^2 =(√2)^2(√3)^22(√2*√3) =232√6 =52√6 Now, 2√6=√24=(approx) So, (√2√3)^2 =52√6 = =(approx) NB The rule \sqrt{ab} = \sqrt{a} \sqrt{b} does not always hold when we extend the \sqrt{

Math Tricks Explained The New York Times

 √3 √2 is rational is wrong Related questions 0 votes 1 answer Prove that root √2 √3 is an irrational number asked in Class X Maths by aditya23 Expert (736k points) 0 votes 1 answer Find two irrational numbers lying The smallest of √8 √5, √7 √6, √10 √3 and √11 √2 is (a) √8 √5 (b) √7 √6 (c) √10 √3 (d) √11 √2 asked in Cubes And2 √ 2,−1 2 √ 2i (Figure 3) −1 2 x y −1 −2 1 u = 1 2 √ 2,−2 √ 2 1 s FIGURE 3 †We assume in Theorems 1 through 5 of this section and their applications that the functions involved have continuous firstorder partial derivatives in open circles

Related » Graph » Number Line » Examples » Our online expert tutors can answer this problem Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Rationalise the denominator in each of the following and hence evaluate by taking √2 = 1414, √3 = 1732 and √5 = 2236 up to three places of decimalStart your free trial In partnership with

 Click here 👆 to get an answer to your question ️ Given that 2√2−√5 / 3√22√5 = =Answer (1 of 227) The answer is 1 1 Explanation Using the rule (ab) (ab) = a^2 b^2 Our equation (2sqrt3) (2sqrt3) = 2^2 sqrt3^2 = 4 3 = 1 Notes 11Rationalise the denominator in each of the following and hence evaluate by taking √2 = 1414, √3 = 1732 and √5 = 2236 up to three places of decimal

Problem 1 Multiples Of 3 And 5 Problem 2 Even Fibonacci Numbers Manualzz

Prove That The Following Are Irrational I 1 2 Ii 7 5 Iii 6 2

Question 3 Categorisation Add an subtract surds that require prior simplification Fully simplify √27√3 wwwdrfrostmathscom Question 4 Categorisation As above but with multiples of surds 53√3 √ Question 7 31−9√2 Question 8 −512√18 Question 9 3 Rationalising denominator of irrational number Add (3√27√3) and (√2−5√3) Divide 5√11 by 3√334 1 i (2 2) = 63i / 5 = 16i To divide complex numbers, you must multiply both (numerator and denominator) by the conjugate of the denominator To find the conjugate of a complex number, you change the sign in imaginary part Distribute in both the numerator and denominator to remove the parenthesis and add and simplify

1

Multiplying And Dividing Radical Expressions

Then use it to find the square root of \sqrt { \frac { 3\sqrt { 5 } }{ 3\sqrt { 5 } } } correct to 2 significant digits asked in Class VIII Maths by priya12 Expert ( 749k points)1 (x 2√2)(x √5) = x² (2√2 √5)x 2√10 = x² 2x√2 x√5 2√10 2 (x² 2x√2 x√5 2√10)(x 3) = x³ 2√2x² √5x² 2√10x 3x² 6√2x 3√5x 6√10 = x³ 3x² 2x²√2 x²√5 6x√2 3x√5 2x√10 6√10 This is not matching any options so we add two more zero's What is the order of √5 , 01, 5/3 , 07, √2 from least to greatest?

Adding And Subtracting With Integers Integers Siyavula

Rd Sharma Solutions Class 9 Maths Chapter 3 Rationalisation Updated For 21 22

In the present case if we multiply the numerator and denominator of the given expression by √3 we get (√5)(√3)/2√3(√3) = √15/2(3) = √15/6 Now the denominator has become rationalAdd 2 / 3 3 / 5 = 2 5 / 3 5 3 3 / 5 3 = 10 / 15 9 / 15 = 10 9 / 15 = 19 / 15 For adding, subtracting, and comparing fractions, it is suitable to adjust both fractions to a common (equal, identical) denominator The common denominator you can calculate as the least common multiple of both denominators LCM(3, 5) = 15In practice, it is enough to find the common denominatorFind the value of √5 correct to 2 decimal places;

Math Tricks Explained The New York Times

Add 2 Root 2 5 Root 3 And Root 2 3 Root 3 Youtube

 answer answered Add 2√2 5√3 and√2 3√3 1 See answer See what the community says and unlock a badge close plus Add answer 5 ptsThere are several ways to go about it Finding The Actual Value Method Here we find the actual value of x, which is nothing but 3 2* (), which is approximately equal to Now we can evaluate the expression (√x1/√x), by taking negative square root for both the parts (√x) and (1/√x)Steps for Solving Linear Equation \frac { x2 } { 3x } \frac { x2 } { 2x } =3 3 x x 2 2 x x − 2 = 3 Variable x cannot be equal to 0 since division by zero is not defined Multiply both sides of the equation by 6x, the least common multiple of 3x,2x Variable x cannot be equal to 0 since division by zero is not defined

Add 2 4 5 And 3 5 6 Youtube

Ex 1 5 2 I Simplify 3 3 2 2 Class 9 With Video

3 3/4 * 2 2/5 The calculator performs basic and advanced operations with fractions, expressions with fractions combined with integers, decimals, and mixed numbers It also shows detailed stepbystep information about the fraction calculation procedure Solve problems with two, three, or more fractions and numbers in one expression Add (3√27√3) and (√2−5√3) (3√27√3) (√2−5√3) = (3√2√2) (7√3 −5√3) = 𝟒√𝟐 𝟐√𝟑 (टीचू) Maths3 b) Add the answer from previous step 9 to the numerator 2 New numerator is 9 2 = 11 c) Write a previous answer (new numerator 11) over the denominator 3 Three and two thirds is eleven thirds Conversion a mixed number 2 1 / 7 to a improper fraction 2 1/7 = 2 1 /

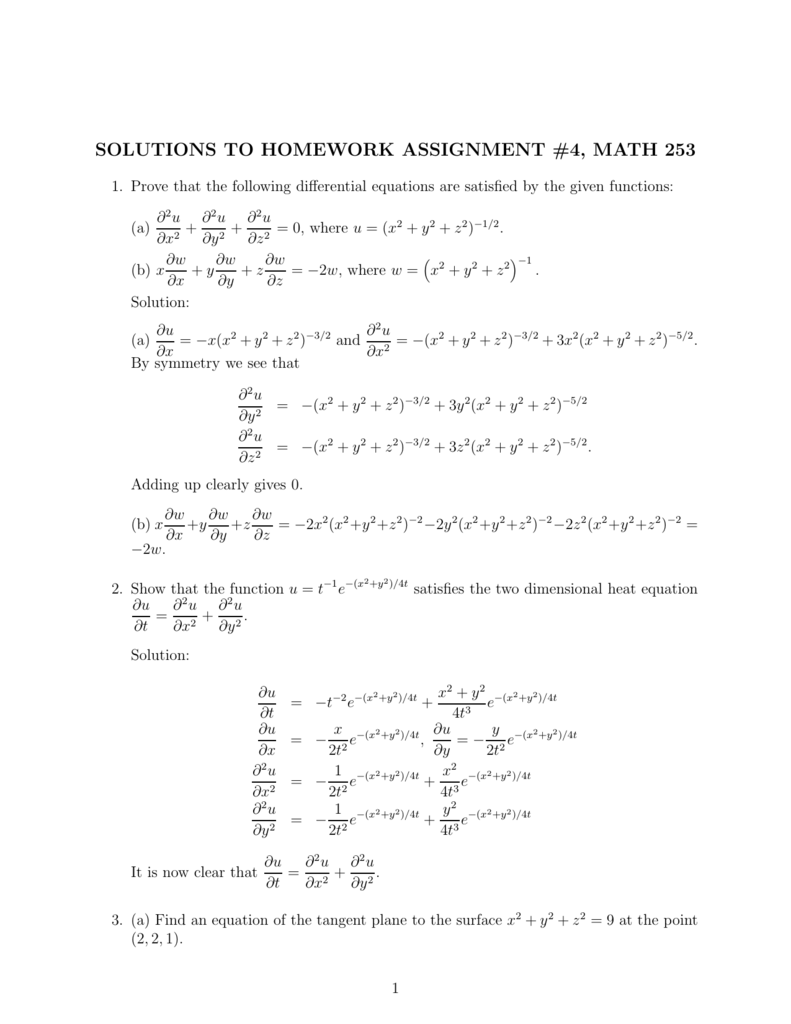
Solutions To Homework Assignment 4 Math 253

Solved 1 Generate A Row Vector X 3 5 4 2 8 9 A Add Chegg Com

Answer (3) 3√3 It is an example of adding two irrational numbers 2√3 √3 We can see that there are two terms that contain two √3, one with coefficient 2 and the other with coefficient 1, and there would be 2 1 = 3 On taking out √3 as common Transcript Simplify (√5√7)^2 (√5√7)^2 =572√35 =𝟏𝟐𝟐√𝟑𝟓 = (√5)^2 (√7)^22√5 √7 About the Author Davneet Singh Davneet Singh is a graduate from Indian Institute of Technology, Kanpur He has been teaching from the past 10 years He provides courses for Maths and Science at Teachoo1 2sqrt(2)1/ 2sqrt2 First multiply bu sqrt(2) ==> 2sqrt(2)*sqrt2 1*sqrt2/2sqrt2*sqrt2 ==> (2*2 sqrt2)/2*2 ==> (4sqrt2)/4 ==> 1sqrt2/4 2 3sqrt2 2sqrt3

How To Solve Surds 2 Four Essential Surd Techniques Suresolv

How To Add And Subtract Square Roots 9 Steps With Pictures

Then we multiply √3 by 2 and √3 by √3 " in above equation" √3×2 = 2√3 √3×√3 = √9 Then we add the above equation ( Solving equation 2) 2√3√9 ( We cannot add this equation because in any number their is a root sign we cannot add it ) At the last we add both the equation 1 and 2 Answer (1 of 3) the value of √3 × √3=(root 3)^2=3 A simple concept can be applied on this question or infact any question of this type ie power adds up when bases are sameY24y1=0 Two solutions were found y =(4√12)/2=2√ 3 = 3732 y =(4√12)/2=2√ 3 = 0268 Step by step solution Step 1 Trying to factor by splitting the middle term

Solved 1 Express The Following Rule In Function Notation Chegg Com

Simplify 4 5 6 2 3 8 3 7 12

 Brainly User = (2√2√2) (5√33√3) = {√2 (21) } {√3 (53) } = { (√2) (3) } {√3 (2) } = 3√2 2√3 bolivianouft and 25 more users found this answer helpful heart outlinedProve that the points (2 a, 4 a), (2 a, 6 a) arid (2a √3, 5a) are the vertices of an equilateral triangle asked in Mathematics by avishek ( 80k points) coordinate geometry Polynomial Division Calculator is a free online tool that shows the division of two polynomials with Polynomial long division method Question 3 If p(x)= x 2 – 2√2x1, then find the value of p(2√2) Solution Put x = 2√2 in p(x), we obtain p(2√2) = (2√2) 2 – 2√2(2√2) 1 = (2√2) 2 – (2√2) 2 1 = 1 Question 4 Find the value of m, if x 4 is a factor of the polynomial

Add 2 5 5 7 3 10 1 5 Mathematics Topperlearning Com Ewwxcpjj

What Is 2 5 2 9

 3√2 7√3 √2 5√3 4√2 2√3 kattyahto8 and 5 more users found this answer helpful heart outlined Thanks 3 star star star starSteps Using Factoring By Grouping 3 { x }^ { 2 } x2 = 0 3 x 2 − x − 2 = 0 To solve the equation, factor the left hand side by grouping First, left hand side needs to be rewritten as 3x^ {2}axbx2 To find a and b, set up a system to be solved To solve the Transcript Question 23 Find a quadratic polynomial whose zeroes are 5 – 3√2 and 5 3√2Given Zeros 5 – 3√2 and 5 3√2 So, Sum of Zeroes = (5 – 3√2) (5 3√2) = 10 Product of Zeroes = (5 – 3√2) × (5 3√2) = 52 − (3√2)2 = 25 − 9 × 2 = 25 − 18 = 7 The Required polynomial is p(x) = x2 − (Sum of Zeroes)x Product of Zeroes = x2 − 10x 7

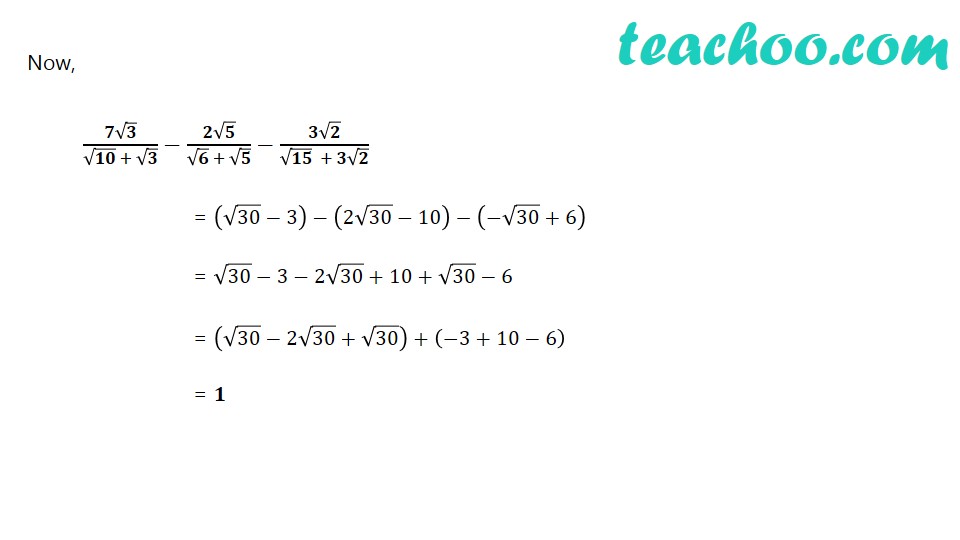
Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 V

Computer Arithmetic Square Root Algorithm By Ali Bohlooli

(ii) (1 i √3) 2 (iii) 4 – 3i (iv) √5 3i Solution (i) 1 – i Given 1 – i We know the multiplicative inverse of a complex number (Z) is Z1 or 1/Z So, ∴ The multiplicative inverse of (1 – i) is (1 i)/2 (ii) (1 i √3) 2 Given (1 i √3) 2 Z = (1 i √3) 2 = 1 2 (i √3) 2 2 (1) (i√3) = 1 3i 2 2 i√3 Example 13 Add 2 √2 5 √3 and √2 3 √3 Here, both numbers have root in it So, we combine the numbers with the same root Combining 2 √2 & √2 and 5√3 & 3 √3, and then solving 2 √2 5 √3 √2 – 3 √3 = (2 √2 √2) (5√3 – 3 If Z = (√3/2i/2)5 (√3/2i/2)5, then (1) Re(Z) = 0 (2) Im(z) = 0 (3) Re(z)>0, Im(z) = 0 (4) Im(z) >0, Im(Z)

What Is 2 3 3 2 5 7 In Descending Order Quora

Wolfram Alpha Examples Step By Step Solutions

The easiest and most boring way to calculate the square root of 2 is to use your calculator!Answer (1 of 6) We know that (ab)(cd)=acadbcbd , √a√a = a and √a√b= √ab (3√5–5√2)(4√53√2) = 12×5 9√10 √10 15×2 = 60 30 9√10 √10 = 30 11√10A 07, √2 , 5/3 , √5 , –01 B √5, √2 , 07, 5/3 , –01

Adding And Subtracting Polynomials

Simplify 8 5 125

Solution Let ω be a primitive 3 −d root of 1, F = Q(ω),E = F(3 √ 2),B = F(3 √ 3) Then by the previous problem H = AutF EB ∼= Z3 ×Z3 H is a subgroup of index 2 in the Galois group G = AutQ EB The complex conjugation σ generates a subgroup of order 2 in G Thus G is a semisirect product of < σ > and H,G = 18 G is theSimply type in 2 followed by √x to get the answer We did that with our calculator and got the following answer with 9 decimal numbers √2 ≈ HowFor example, if we add two irrational numbers, say 3 √2 4√3, a sum is an irrational number But, let us consider another example, (34√2) (4√2 ), the sum is 3, which is a rational number So, we should be very careful while adding and multiplying two irrational numbers, because it might result in an irrational number or a rational

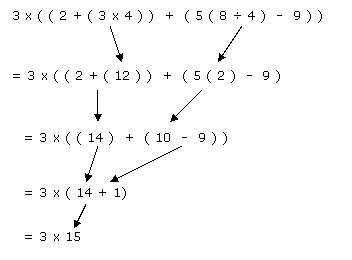
Order Of Operations

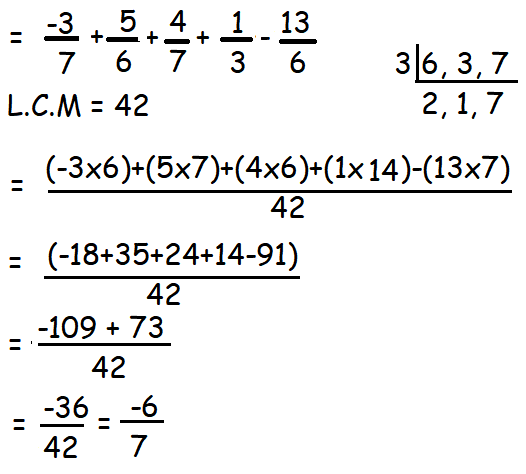
Using Mean Method To Find Rational Numbers Between Two Numbers

2 X∞ n=1 cos2(n) √ n3 Solution Since 0 ≤ cos2(n) √ n3 ≤ 1 n3 2, and X∞ n=0 1 n3 2 converges by pseries test (p = 3 2 >1), then comparison test yields the convergence of X∞ n=1 cos2(n) √ n3 b 6 points Decide whether each of the following series converges absolutely, converges conditionally or diverges Circle your answer

Add 3 2 7 3 And 2 5 3 Brainly In

Simplify Add Or Subtract Fractions With Step By Step Math Problem Solver

Worksheet 10

Cbse 9 Math Cbse Number Systems Ncert Solutions

If 2 3 8 3 7 27 4 5 32 5 8 60 6 7 72 Then The Value Of 7 8 Is Quora

Addition And Subtraction Of Surds Msrblog

Adding And Subtracting Radical Expressions

How To Add And Subtract Square Roots 9 Steps With Pictures

Square Root Of 2 Wikipedia

Find The Values Of A And B If 7 3 5 3 5 7 3 5 3 5

Multiplying And Dividing Radical Expressions

Bodmas Rule What Is Bodmas Rule Order Of Operations With Examples

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 Sarthaks Econnect Largest Online Education Community

Add 2 Root 2 5 Root 3 And Root 2 3 Root 3 2 2 5 3 And 2 3 3 Youtube

Nnzrnmfkryualm

Example 13 Add 2 Root2 5 Root3 And Root2 3 Root3 Examples

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 V

Chapter 7 Radicals Radical Functions And Rational Exponents Ppt Video Online Download

Rationalize The Denominator And Simplify I 3 2 3 2 Ii 5 2 3 7 4 3 Sarthaks Econnect Largest Online Education Community

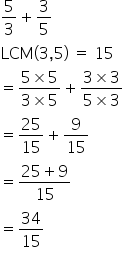
Find The Sum Of 5 3 And 3 5 Mathematics Topperlearning Com Igisa6ll

Cbse 9 Math Cbse Number Systems Ncert Solutions

Add 5root 2 3 Root 3 And 2 Root 2 5root3 Brainly In

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 Studyrankersonline

How To Add And Subtract Square Roots 9 Steps With Pictures

How To Add And Subtract Square Roots 9 Steps With Pictures

Rationalize The Denominator Meaning Methods Examples

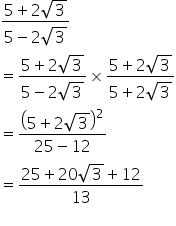
5 2 3 5 2 3 Mathematics Topperlearning Com Rvsvlgmm

Simplifying By Rationalising The Denominator 3 5 3 2 5 3 Studyrankersonline

Add 2 Squareroot 2 5 Squareroot 3 And Square Root2 Square Root 3 Brainly In

Document

Rationalise The Denominator Of 5 3 5 3 Studyrankersonline

How To Add And Subtract Square Roots 9 Steps With Pictures

Ncert Exemplar Class 9 Maths Solutions Chapter 1 Number Systems Cbse Tuts

Simplifying Square Root Expressions No Variables Video Khan Academy

Multiplying Complex Numbers Video Khan Academy

Ways To Solve Quadratic Equations Algebra I Discriminant En Equation Factoring Math Ways To Solve Quadra Glogster Edu Interactive Multimedia Posters

Multiply 3 Root 5 By 2 Root 6 Root 15 By 4 Root 5 2 Root 6 By 3 Root 3 3 Root 8 By 3 Root 2 Youtube

Problem Solving If 3 2 7 5 4 23 7 6 47 And 9 8 79 To What Is 10 9 Equal Quora

Solved Add 1 2 7 10 Subtract 8 1 4 6 Multiply 2 Chegg Com

Assuming That X Y Z Are Positive Real Numbers Simplify The 2 3 5 6 7 2 Sarthaks Econnect Largest Online Education Community

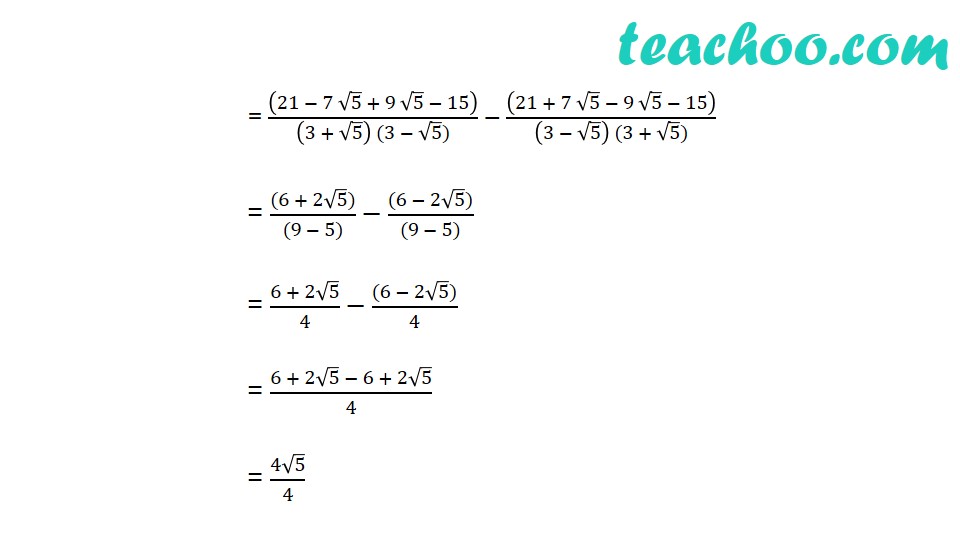
Find The Values Of A And B If 7 3 5 3 5 7 3 5 3 5

Fractions Addition Calculator

Add 2 3 5 2 And 3 2 2 Brainly In

True Or False Nz Maths

Rationalize 2 3 5 2 2 3 3 Brainly In

Simplify I 5 3 8 3 Ii 9 5 4 5 125 Iii 7 48 27 3 Iv 7 3 5 7 2 7 Sarthaks Econnect Largest Online Education Community

Rd Sharma Solutions Class 9 Maths Chapter 3 Rationalisation Updated For 21 22

Directions Add Or Subtract The Radical Expression Gauthmath

Ml Aggarwal Solutions For Class 9 Chapter 1 Rational And Irrational Numbers Avail Free Pdf

1

Surds What Is A Surd Manipulating And Simplifying Surds Ppt Download

Msm08g8ga Tg I Nbsp Sbquo 4e Squares And Square Roots Name Date Class Homework And Practice 4e Squares And Square Roots 8 Sample Use The Square Root Of The

R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3

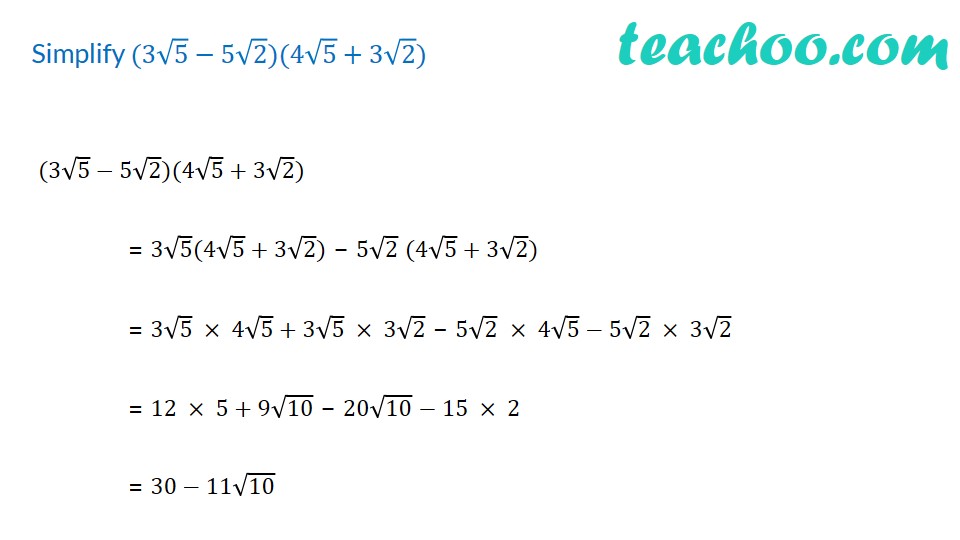
Simplify 3 5 5 2 4 5 3 2 With Video Teachoo Rationalising

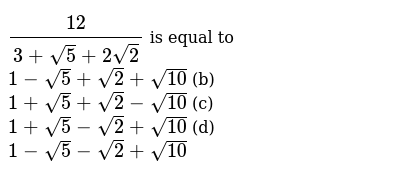
12 3 Sqrt 5 2sqrt 2 Is Equal To 1 Sqrt 5 Sqrt 2 Sqrt 10 B 1 Sqrt 5 Sqrt 2 Sqrt 10 C 1 Sqrt 5 Sqrt 2 Sqrt 10 D 1 Sqrt 5 Sqrt 2 Sqrt 10

Surds Mathematics Gcse Revision

Solve A 1 18 1 18 B 8 15 3 15 C 7 5 5 7 D 1 22 21 22 E 12 15 7 15 F 5 8 3 8 G 1 2 3 1 3 3 H 1 4 0 4 I 3 12 5

Add 3 2 7 3 And 2 5 3 Brainly In

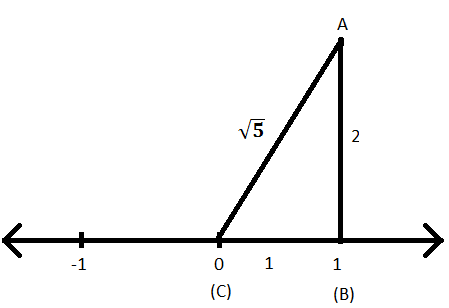
Representation Of Irrational Numbers On The Number Line 2 On Number Line

Add 2 3 5 5 7 7 And 3 5 3 7 Brainly In

If 4 3 5 4 3 5 A B 5 Then What Are The Values Of A And B

Add 2 3 5 2 And 3 2 2 2 2 5 3 7 5 And 3 3 2 5 2 3 7 1 2 2 6 11 Youtube

Add 2 4 5 And 3 5 6 Youtube

Expert Verified 5 2 3 7 4 3 A B 3 Find The Value Of A And B Of The Following Brainly In

Simplify By Rationalising The Denominator 30 5 3 3 5 Studyrankersonline

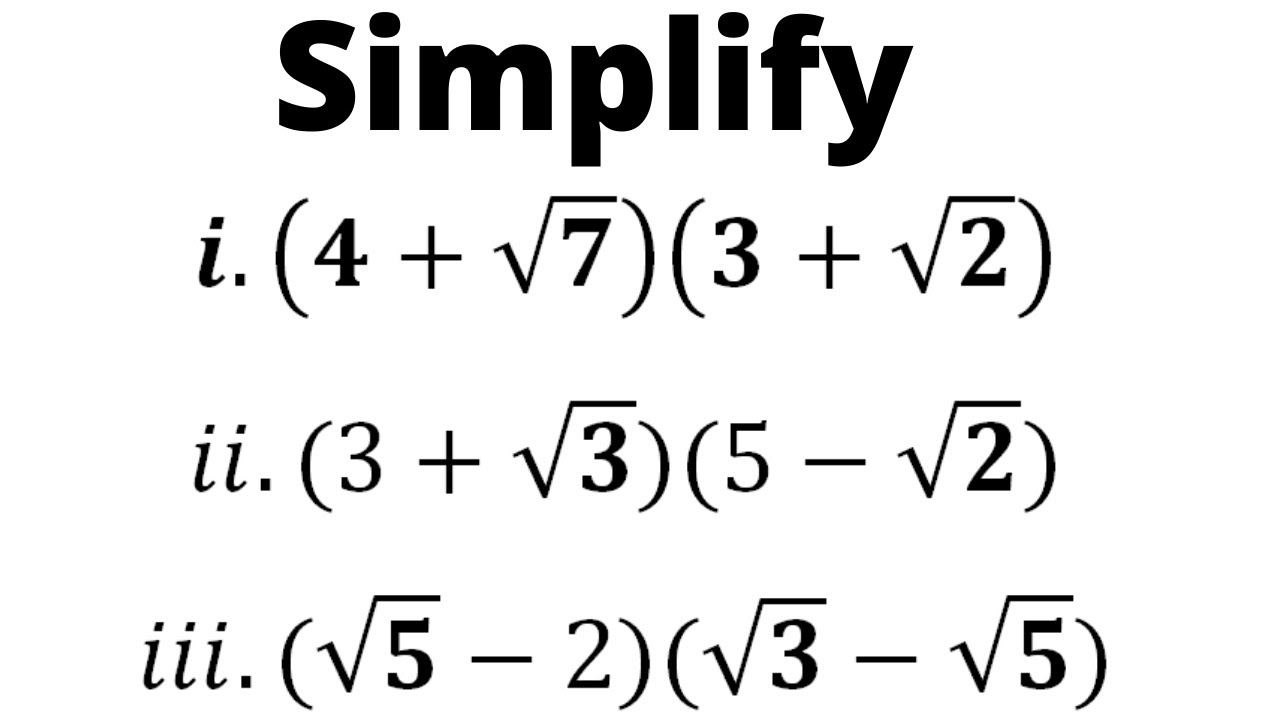
Simplify I 4 Root 7 3 Root 2 Ii 3 Root 3 5 Root 2 Iii Root 5 2 Root 3 Root 5 Youtube

What Is 2 3 5 6

Adding Subtracting Radicals Square Roots Purplemath

Adding Fractions

Answer Page 1

Add And Subtract Square Roots Elementary Algebra

R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3

Find The Sum Of 3 Root 3 7 Root 2 And Root 3 5 Root 2 Studyrankersonline

How To Calculate 2 3 2 Quora

R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3

How To Sum 1 1 2 3 1 2 3 4 1 3 4 5 For Up To 10 Terms Quora

2 2

What Is The Answer Of Simplify 7 3 5 3 5 7 3 5 3 5 Quora

Incoming Term: add 2 root 3 - 5 root 2 and root 3 + 2 root 2,